淺談重度視障者的數學讀寫方式
說明
-
數學的特性
樹狀結構:每個物件均可延伸
-
點字的特性
線形結構:單行呈現
-
範例
-
分數
-
視覺 \(\frac{1}{2}\)
-
數學點字 ⠹⠂⠌⠆⠼
-
指數
-
視覺 \(5^{3+2}\)
-
數學點字 ⠼⠢⠘⠒⠬⠆
-
根號
-
視覺 \(\sqrt{a+b}\)
-
數學點字 ⠜⠁⠬⠃⠻
-
混合
-
視覺 \(x=\frac{-b \pm \sqrt{b^2-4ac}}{2a}\)
-
數學點字 ⠭⠀⠨⠅⠀⠹⠤⠃⠬⠤⠜⠃⠘⠆⠐⠤⠲⠁⠉⠻⠌⠆⠁⠼
-
如何讓視障者與明眼人溝通數學?
讀寫媒介
-
紙本
-
點字機、點字板、點字印表機
-
優點:摸讀時可前後快速對照
-
缺點:紙本整理不易,互動不易
-
電腦
-
螢幕報讀軟體、點字觸摸顯示器
-
優點:互動方便、及時
-
缺點:訊息為線形呈現
讀寫語法
|
算式 |
語法 |
|
\(\frac{\sqrt{3}+1}{2}\) |
⠹⠜⠒⠻⠬⠂⠌⠆⠼ |
|
\(\frac{\sqrt{3+1}}{2}\) |
⠹⠜⠒⠬⠂⠻⠌⠆⠼ |
|
\(\frac{\sqrt{3}}{2}+1\) |
⠹⠜⠒⠻⠌⠆⠼⠬⠂ |
|
\([(-8)\times6-3]+|(-5)\times10-1|\) |
⠈⠷⠷⠤⠦⠾⠈⠡⠖⠤⠒⠈⠾⠬⠳⠷⠤⠢⠾⠈⠡⠂⠴⠤⠂⠳ |
|
算式 |
語法 |
|
\(\frac{\sqrt{3}+1}{2}\) |
\frac{\sqrt{3}+1}{2} |
|
\(\frac{\sqrt{3+1}}{2}\) |
\frac{\sqrt{3+1}}{2} |
|
\(\frac{\sqrt{3}}{2}+1\) |
\frac{\sqrt{3}}{2}+1 |
|
\([(-8)\times6-3]+|(-5)\times10-1|\) |
[(-8)\times6-3]+|(-5)\times10-1| |
語法比較
分數
|
類型 |
語法 |
|
視覺 |
\(\frac{1}{2}\) |
Nemeth
數學點字 |
⠹⠂⠌⠆⠼
?1/2# |
|
LaTeX |
\frac{1}{2} |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<mfrac>
<mn>1</mn>
<mn>2</mn>
</mfrac>
</math>
|
根號
|
類型 |
語法 |
|
視覺 |
\(\sqrt{2}\) |
Nemeth
數學點字 |
⠜⠆⠻
>2} |
|
LaTeX |
\sqrt{2} |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<msqrt>
<mn>2</mn>
</msqrt>
</math>
|
指數
|
類型 |
語法 |
|
視覺 |
\(5^2\) |
Nemeth
數學點字 |
⠼⠢⠘⠆
#5~2 |
|
LaTeX |
5^2 |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<msup>
<mn>5</mn>
<mn>2</mn>
</msup>
</math>
|
混合
|
類型 |
語法 |
|
視覺 |
\(x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\) |
Nemeth
數學點字 |
⠭⠀⠨⠅⠀⠹⠤⠃⠬⠤⠜⠃⠘⠆⠐⠤⠲⠁⠉⠻⠌⠆⠁⠼
x .k ?-b+->b~2"-4ac}/2a# |
|
LaTeX |
x=\frac{-b\pm \sqrt{b^2-4ac}}{2a} |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<mi>x</mi>
<mo>=</mo>
<mfrac>
<mrow>
<mo>−<!-- − --></mo>
<mi>b</mi>
<mo>±<!-- ± --></mo>
<msqrt>
<msup>
<mi>b</mi>
<mn>2</mn>
</msup>
<mo>−<!-- − --></mo>
<mn>4</mn>
<mi>a</mi>
<mi>c</mi>
</msqrt>
</mrow>
<mrow>
<mn>2</mn>
<mi>a</mi>
</mrow>
</mfrac>
</math>
|
聯立方程式
|
類型 |
語法 |
|
視覺 |
\(\begin{cases}x+y=6\\x-y=1\end{cases}\) |
Nemeth
數學點字 |
⠨⠠⠷⠭⠬⠽⠀⠨⠅⠀⠼⠖
⠨⠠⠷⠭⠤⠽⠀⠨⠅⠀⠼⠂
.,(x+y .k #6
.,(x-y .k #1 |
|
LaTeX |
\begin{cases}
x+y=6\\
x-y=1
\end{cases}
|
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<mrow>
<mo>{</mo>
<mtable>
<mtr>
<mtd>
<mi>x</mi>
<mo>+</mo>
<mi>y</mi>
<mo>=</mo>
<mn>6</mn>
</mtd>
</mtr>
<mtr>
<mtd>
<mi>x</mi>
<mo>−<!-- − --></mo>
<mi>y</mi>
<mo>=</mo>
<mn>1</mn>
</mtd>
</mtr>
</mtable>
<mo fence="true" stretchy="true" symmetric="true"></mo>
</mrow>
</math>
|
圖形記號
|
類型 |
語法 |
|
視覺 |
\(\triangle{ABC} \cong \triangle{DEF}\) |
Nemeth
數學點字 |
⠫⠞⠠⠁⠠⠃⠠⠉⠀⠈⠱⠨⠅⠀⠫⠞⠀⠠⠙⠠⠑⠠⠋
$t ,a,b,c `:.k $t ,d,e,f |
|
LaTeX |
\triangle{ABC} \cong \triangle{DEF} |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<mi mathvariant="normal">△<!-- △ --></mi>
<mrow class="MJX-TeXAtom-ORD">
<mi>A</mi>
<mi>B</mi>
<mi>C</mi>
</mrow>
<mo>≅<!-- ≅ --></mo>
<mi mathvariant="normal">△<!-- △ --></mi>
<mrow class="MJX-TeXAtom-ORD">
<mi>D</mi>
<mi>E</mi>
<mi>F</mi>
</mrow>
</math>
|
線段
|
類型 |
語法 |
|
視覺 |
\(\overline{AB} \perp \overline{CD}\) |
Nemeth
數學點字 |
⠐⠠⠁⠠⠃⠣⠱⠻⠀⠫⠏⠀⠐⠠⠉⠠⠙⠣⠱⠻
",a,b<:} $p ",c,d<:} |
|
LaTeX |
\overline{AB} \perp \overline{CD} |
|
MathML |
<math xmlns="http://www.w3.org/1998/Math/MathML">
<mover>
<mrow>
<mi>A</mi>
<mi>B</mi>
</mrow>
<mo accent="false">¯</mo>
</mover>
<mo>⊥</mo>
<mover>
<mrow>
<mi>C</mi>
<mi>D</mi>
</mrow>
<mo accent="false">¯</mo>
</mover>
</math>
|
結論
視覺障礙輔助科技已發展至成熟階段,在數學學習上,除了圖表之外,幾乎可完全依賴電腦來學習,而上述可用的數學書寫紀錄語法中,考量學習的難易度,以及使用範圍的大小,建議:
-
在中小學數學概念建構的初期,較適合用簡單的 Nemeth 數學點字來計算與書寫
-
進入高中及大學,如果是讀理組的,則可開始接觸 LaTeX 或 MathML
當然這些語法要表示的其實都是同一種東西,如果可以在彼此間互轉,是最理想的狀態。
解決方案:
操作範例
感謝 鄭明芳 老師開發好用的軟體,讓點字使用者可以和一般人進行數學互動!
數學點字轉 MathML 數學式
新課綱的計算機議題
計算機融入數學學習
新課綱課本內容(截圖自康軒數學一上 1-3 P.61)
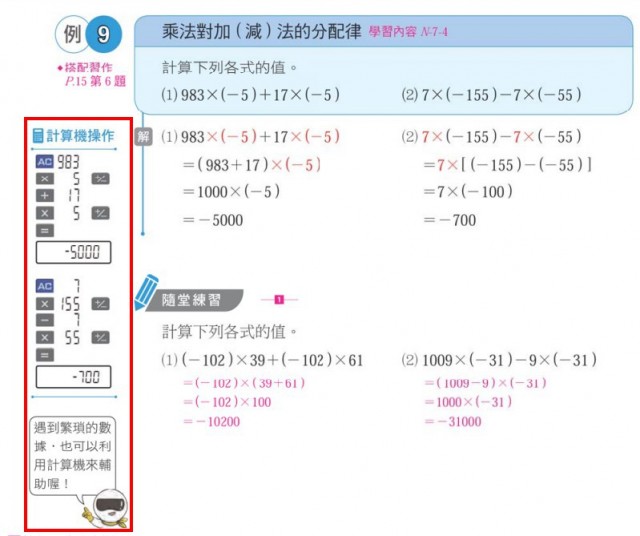
解決方案:





